
X 2 X 1 0, , , , , , , 0, Example 10 - Solve x2 + x + 1 = 0 - Chapter 5 NCERT - Examples, www.teachoo.com, 945 x 945, jpeg, x2 solve chapter example, 20, x-2-x-1-0, KAMPION
WebGCF of 9 and 18 is the divisor that we get when the remainder becomes 0 after doing long division repeatedly. Step 1: Divide 18 (larger number) by 9 (smaller number). Step 2:. WebThe second method to find GCF for numbers 9 and 18 is to list all Prime Factors for both numbers and multiply the common ones: All Prime Factors of 9: 3, 3. All Prime Factors of. WebThere are multiple ways to find the greatest common factor of given integers. One of these involves computing the prime factorizations of each integer, determining which factors.
Web9 = 3 × 3. 18 = 2 × 3 × 3. The common prime factors of 9 and 18 are 3 and 3. GCF is equal to the multiplication of those prime factors. GCF ( 9, 18) = 3 × 3 = 9. WebHow to Find the GCF of 9 and 18? Answer: Greatest Common Factor of 9 and 18 = 9. Step 1: Find the prime factorization of 9. 9 = 3 x 3. Step 2: Find the prime factorization of 18. 18. Web9 = 3 × 3. Find the prime factorization of 18. 18 = 2 × 3 × 3. To find the GCF, multiply all the prime factors common to both numbers: Therefore, GCF = 3 × 3. GCF = 9. MathStep. WebAnswer (1 of 4): Q: The GCF of 18 and n is 9. What are the possible values of n if it’s a two digit number? The greatest factor of 18, other than 18 itself, is 9. Therefore we only need. WebPrime factors of 72 are: 2, 2, 2, 3, 3, Prime factors of 40 are: 2, 2, 2, 5, In other words, we can write: 72 = 2 * 2 * 2 * 3 * 3 and 40 = 2 * 2 * 2 * 5, The part which is. WebWhat is the GCF of 18 and 9? Asked by Wiki User. See Answer. Top Answer. Wiki User Answered . 2017-11-15 12:18:42. The GCF is 9. 0 0 1. WebGCF of 18 and 90 is the divisor that we get when the remainder becomes 0 after doing long division repeatedly. Step 1: Divide 90 (larger number) by 18 (smaller number). Step 2:. WebGCF of 18 and 9 is 9. This answer is: Wiki User. ∙ 2017-11-11 14:08:39. Copy. GCF (18, 9) = 9. This answer is: WebFor instance, let us find the gcf(9,18). In this case we have: The factors of 9 (all the whole numbers that can divide the number without a remainder) are 1, 3 and 9; The factors of.
GCF of 9 and 18 | How to Find GCF of 9, 18?

GCF of 18 and 36-How to find GCF of 18 and 36
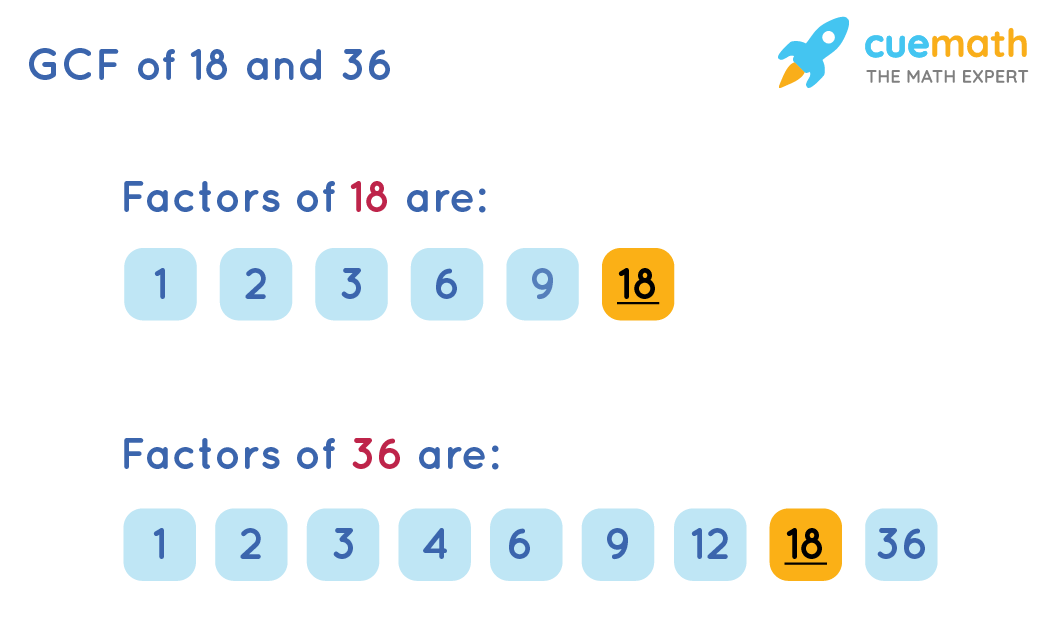
3 3
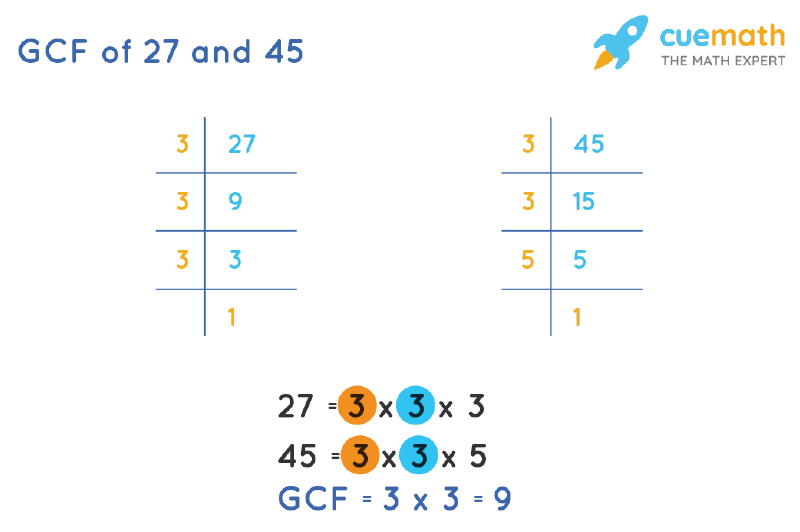
GCF of 27 and 45 | How to Find GCF of 27, 45?
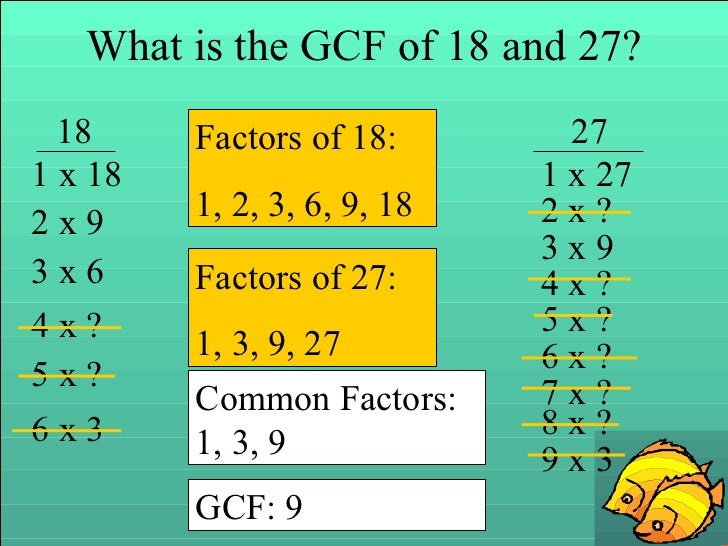
How To Find The GCF (Greatest Comon Factor) and LCM (Least Common
38+ Gcf Of 30 Most Popular - Volume
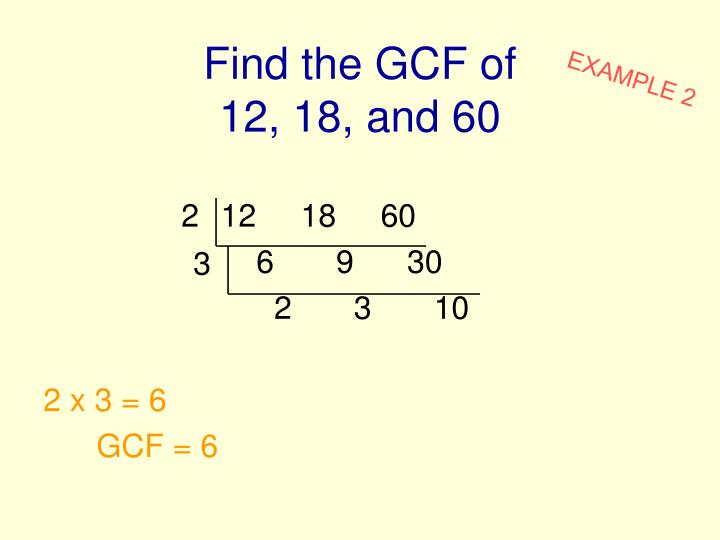
Greatest Common Factor (solutions, examples, videos)
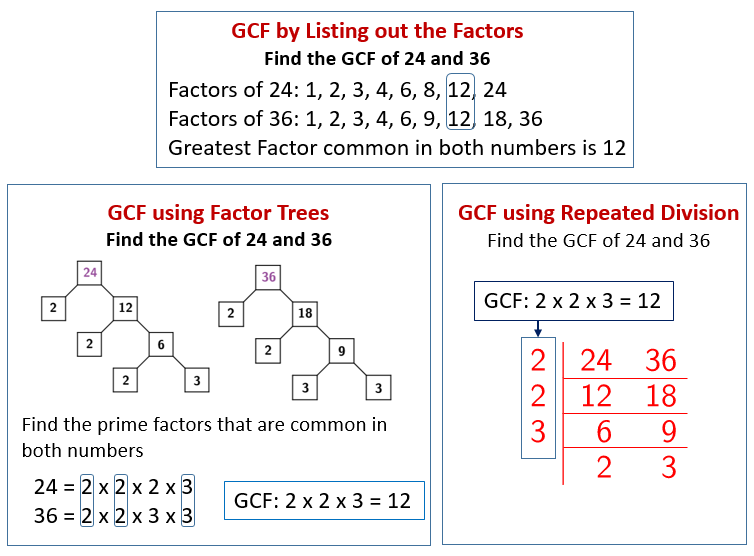
PPT - Greatest Common Factor (GCF) PowerPoint Presentation, free
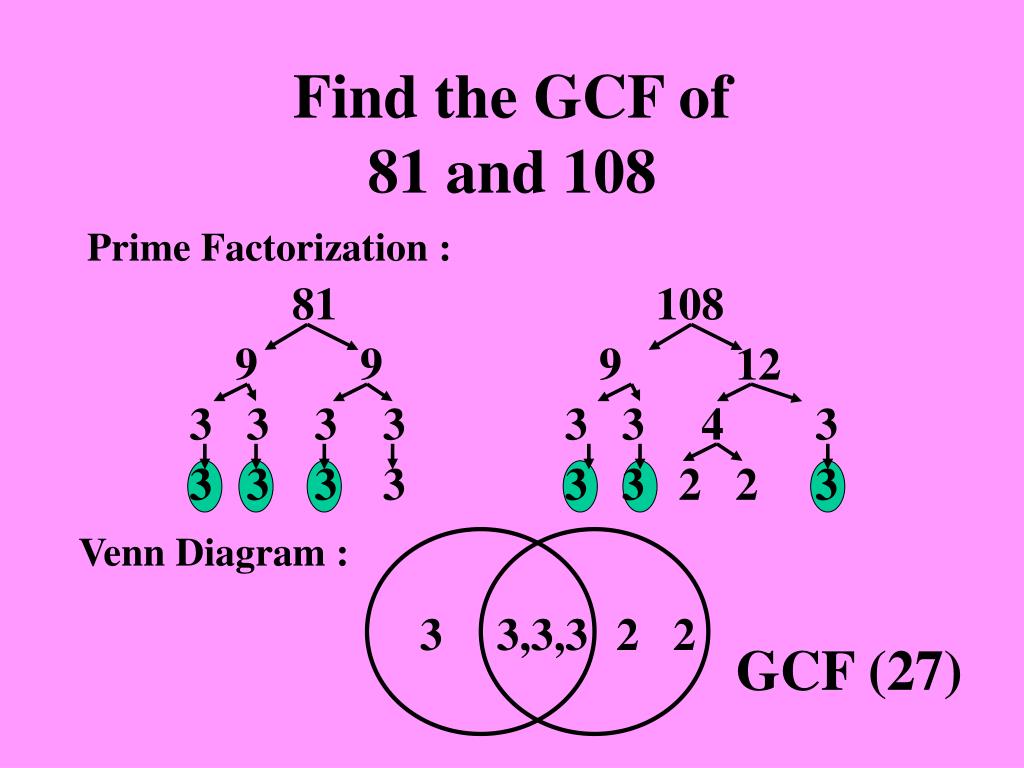
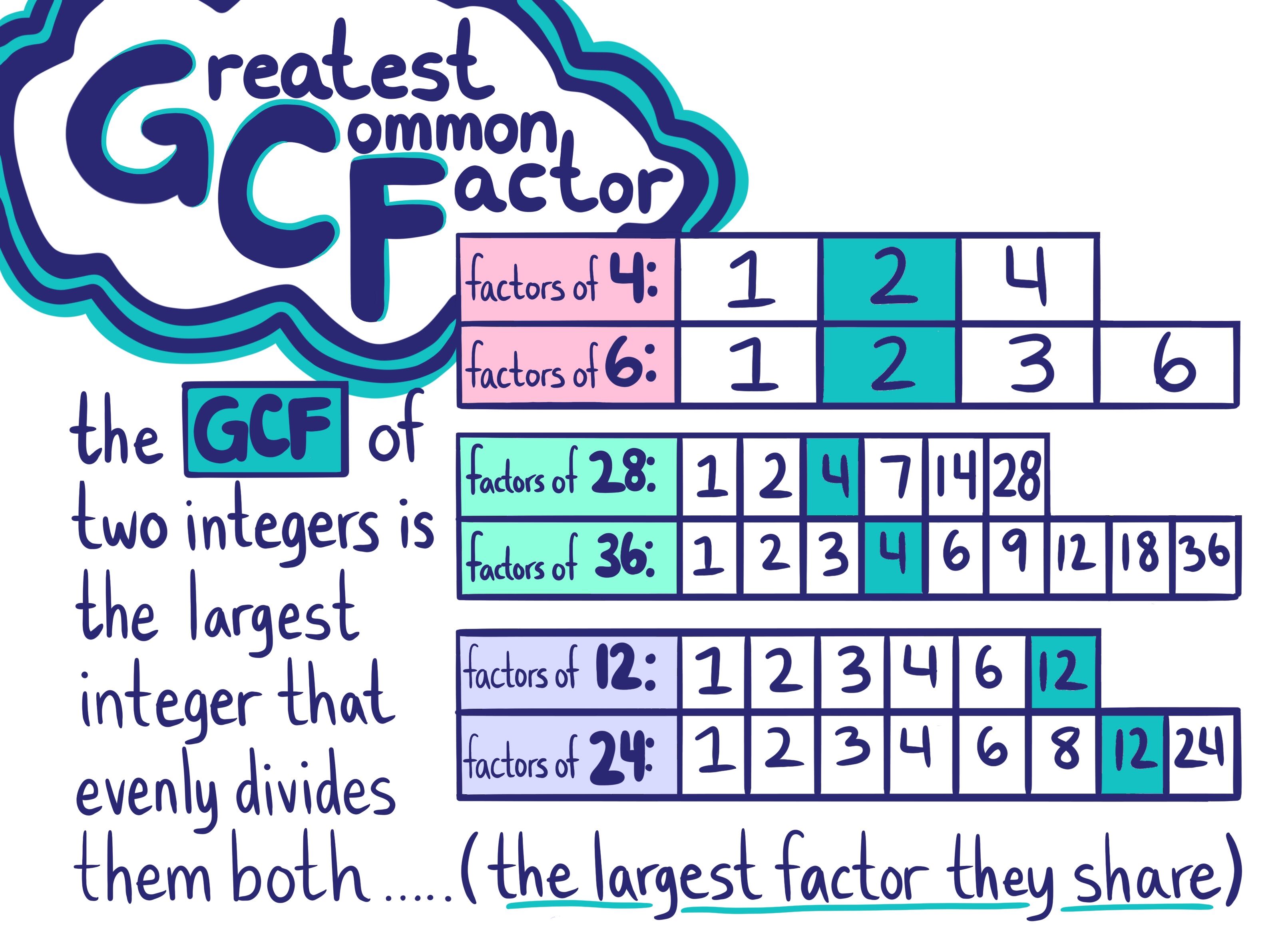
Greatest Common Factor (GCF) – Definition, Formula, Examples | How to
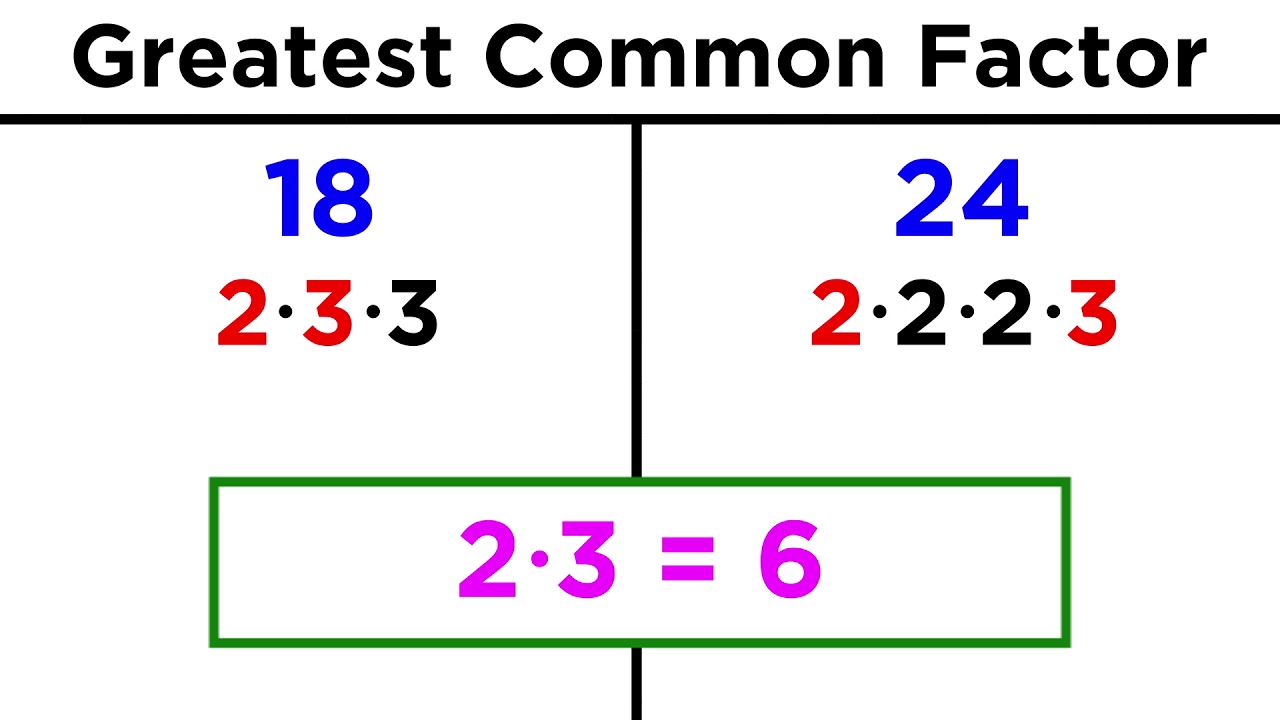
Greatest Common Factor (GCF) - Expii
Tidak ada komentar:
Posting Komentar